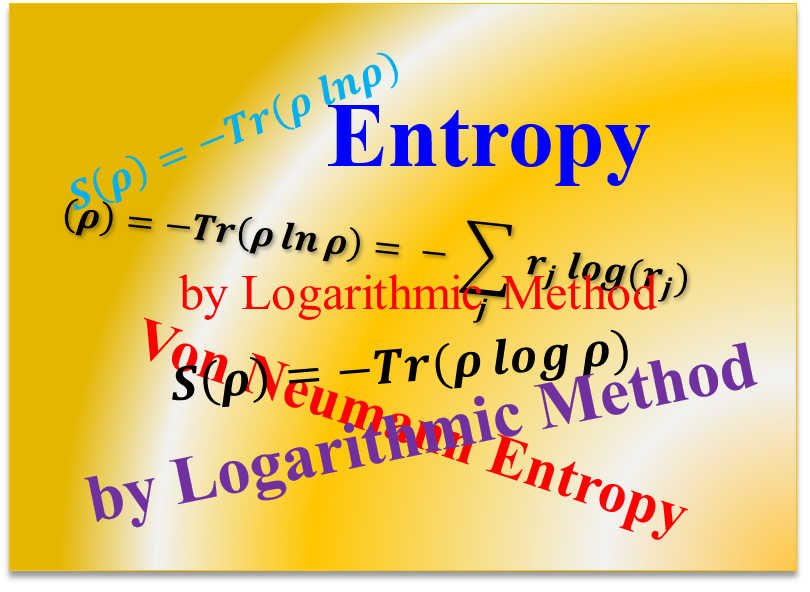
Von Neumann Entropy by Logarithmic Method
Mathematics-Physics
DOI:
https://doi.org/10.14331/ijfps.2019.330132Keywords:
Entropy, Von Neumann entropy, linearityAbstract
The Von Neumann entropy plays a central role in the quantum information theory and is a concave function and following the property . In this paper, we introduce a new proof for the linearity of Von Neumann entropy in the rate without using the above inequality. Here the Von Neumann entropy is concave; that is, given weights and density matrices . Roughly speaking, we will show that in the rate case, the Von Neumann entropy is linear without using Fannes inequality.
Downloads
Author Biography
Bijan Nikouravan, Department of Physics, Islamic Azad University (IAU),Varamin-Pishva Branch, Iran
References
Audenaert, K. M. (2007). A sharp continuity estimate for the von Neumann entropy. Journal of Physics A: Mathematical and Theoretical, 40(28), 8127.
Garbaczewski, P. (2005). Differential entropy and time. Entropy, 7(4), 253-299.
Jaeger, G. (2007). Quantum information: Springer.
Jaynes, E. T. (1965). Gibbs vs Boltzmann entropies. American Journal of Physics, 33(5), 391-398.
Kullback, S. (1968). Information Theory and Statistics. Courier Corporation. image analysis. Composites Science and Technology, 59(4), 543-545.
Neumann, J. v. (2013). Mathematische grundlagen der quantenmechanik (Vol. 38): Springer-Verlag.
Nielsen, M. A., & Chuang, I. L. (2000). Quantum information and quantum computation. Cambridge: Cambridge University Press, 2(8), 23.
Shannon, C. E. (1948). A mathematical theory of communication. Bell system technical journal, 27(3), 379-423.
Tomamichel, M., Colbeck, R., & Renner, R. (2010). The duality between smooth min-and max-entropies. IEEE Transactions on information theory, 56(9), 4674-4681.
von NEUMANN, J., & BEYER, R. T. (1955). Mathematische Grundlagen der Quantenmechanik. Mathematical Foundations of Quantum Mechanics... Translated... by Robert T. Beyer: Princeton University Press.
Published
How to Cite
Issue
Section
License
Copyright (c) 2019 International Journal of Fundamental Physical Science
This work is licensed under a Creative Commons Attribution 4.0 International License.
